International Terrestrial Reference Frame 2014 (ITRF2014)

On this page:

ITRF definitions

An International Terrestrial Reference Frame (ITRF) is a realization of the International Terrestrial Reference System (ITRS), maintained by the International Earth Rotation and Reference Systems Service (IERS). Official website: itrf.ensg.ign.fr.

A Terrestrial Reference System (TRS) is a spatial reference system co-rotating with the Earth in its diurnal motion in space. The ITRS imposes a no net rotation (NNR) condition for horizontal motions which means that the datum is not tied to any specific tectonic plate. In such a system, positions of points anchored on the Earth solid surface have coordinates which undergo only small variations with time, due to geophysical effects (tectonic or tidal deformations). A Terrestrial Reference Frame (TRF) is a set of physical points with precisely determined coordinates in a specific coordinate system (cartesian, geographic, mapping...) attached to a Terrestrial Reference System. Such a TRF is said to be a realization of the TRS.

The ITRF solutions do not directly use an ellipsoid. ITRF solutions are specified by cartesian ECEF (Earth-Centered, Earth-Fixed) coordinates X, Y, and Z. If needed they can be transformed to geographical coordinates (Longitude, Latitude and Height) referred to an ellipsoid. In this case the GRS80 ellipsoid is recommended (semi-major axis a=6378137.0 m, flattening=1/298.257222101). This ellipsoid was adopted at the XVII General Assembly of the International Union of Geodesy and Geophysics (IUGG). The GRS80 reference system was originally used by the World Geodetic System 1984 (WGS84). The reference ellipsoid of WGS84 now differs slightly due to its later refinements.

The current realization of the ITRS is the ITRF2014 solution, published by the IERS on 22 January 2016. The ITRF2014 solution replaces the ITRF2008 solution that was published by the IERS on 31 May 2010. ITRF2014 consists of sets of station positions and velocities with their variance/covariance matrices. It has been computed using solutions from four difference space geodetic techniques: VLBI (Very Long Baseline Interferometry), SLR (Satellite Laser Ranging), DORIS (Doppler Orbitography and Radiopositioning Integrated by Satellite), and GPS (Global Positioning System). Technique centres: International VLBI Service for Geodesy and Astrometry (IVS; ivscc.gsfc.nasa.gov), International Laser Ranging Service (ILRS; ilrs.gsfc.nasa.gov), International DORIS Service (IDS; ids-doris.org), and the International GNSS Service (IGS; igscb.jpl.nasa.gov).

GRS80 parameters

IERS recommends to use the Geodetic Reference System 1980 (GRS80) ellipsoid as its reference ellipsoid with the geometric center of the ellipsoid coincident with the center of mass of the Earth and the origin of the coordinate system.

Parameter	Notation	Value
Semi-major Axis	a	6378137.0 m
Flattening Factor of the Earth	1/f	298.257222101

The difference between the GRS80 and WGS84 values for f creates a difference of 0.1 mm in the derived semi-minor axes of the two ellipsoids.

ITRF realizations

Both the EPSG database and the IERS website use 'ITRF2014' without spaces between 'ITRF' and '2014'.

CRS Code	Datum Code	Short Name	Datum Epoch	Area Code	Area Name	Remarks
4978	6326	WGS84	1984	1262	World	No distinction is made in the EPSG database between the original WGS84 frame, WGS84 (G730), WGS84 (G873), WGS84 (G1150) and WGS84 (G1674). Since 1997, WGS84 has been maintained within 10 cm of the then current ITRF.
4910	6647	ITRF88	1984.0	1262	World	Origin at geocentre, orientated to the BIH Terrestrial System at epoch 1984.0. Datum defined by a set of 3-dimensional Cartesian station coordinates (SCS).
4911	6648	ITRF89	1984.0	1262	World	Origin at geocentre, orientated to the BIH Terrestrial System at epoch 1984.0. Datum defined by a set of 3-dimensional Cartesian station coordinates (SCS).
4912	6649	ITRF90	1984.0	1262	World	Origin at geocentre, orientated to the BIH Terrestrial System at epoch 1984.0. Datum defined by a set of 3-dimensional Cartesian station coordinates (SCS). Parameters from ITRF90 to WGS84-Doppler realized system: WGS84.TXT
4913	6650	ITRF91	1984.0	1262	World	Origin at geocentre, orientated to the BIH Terrestrial System at epoch 1984.0. Datum defined by a set of 3-dimensional Cartesian station coordinates (SCS).
4914	6651	ITRF92	1984.0	1262	World	Origin at geocentre, orientated to the BIH Terrestrial System at epoch 1984.0. Datum defined by a set of 3-dimensional Cartesian station coordinates (SCS).
4915	6652	ITRF93	1984.0	1262	World	Origin at geocentre, orientated to the BIH Terrestrial System at epoch 1984.0. Datum defined by a set of 3-dimensional Cartesian station coordinates (SCS).
4916	6653	ITRF94	1984.0	1262	World	Origin at geocentre, orientated to the BIH Terrestrial System at epoch 1984.0. Datum defined by a set of 3-dimensional Cartesian station coordinates (SCS).
4917	6654	ITRF96	1984.0	1262	World	Origin at geocentre, orientated to the BIH Terrestrial System at epoch 1984.0. Datum defined by a set of 3-dimensional Cartesian station coordinates (SCS).
4918	6655	ITRF97	1984.0	1262	World	Origin at geocentre, orientated to the BIH Terrestrial System at epoch 1984.0. Datum defined by a set of 3-dimensional Cartesian station coordinates (SCS).
4919	6656	ITRF2000	1997.0	1262	World	Origin at geocentre, orientated to the BIH Terrestrial System at epoch 1984.0. Datum defined by a set of 3-dimensional Cartesian station coordinates (SCS).
4896	6896	ITRF2005	2000.0	1262	World	Origin at geocentre, originally orientated to the BIH Terrestrial System at epoch 1984.0, then adjusted to ensure a zero net rotation connection to earth's overall tectonic motion. Defined by time series of Cartesian station coordinates and Earth Rotation parameters. Transformation parameters between ITRF2005 and ITRF2000: tp_05-00.php
5332	1061	ITRF2008	2005.0	2830	World	Origin at geocentre. The origin is defined in such a way that there are null translation parameters at epoch 2005.0 and null translation rates between the ITRF2008 and the ILRS SLR time series. Transformation parameters between ITRF2005 and ITRF2008: tp_08-05.php
7789	1165	ITRF2014	2010.0	2830	World	Origin at geocentre. The origin is defined in such a way that there are zero translation parameters at epoch 2010.0 and zero translation rates between the ITRF2014 and the ILRS SLR time series. Transformation parameters between ITRF2005 and ITRF2008: tp_14-08.php
		ITRF2020

ITRF and WGS84

In general the ITRS (and its realizations ITRFyy) are identical to WGS84 at one meter level. Meanwhile there are two types of WGS84 realization.

Old realization based on U.S. Navy Navigation Satellite System, commonly known as DOPPLER Transit, and provided station coordinates with accuracies of about one meter.
With respect to this realization the International Earth Rotation Service published transformation parameters between ITRF90 and this Doppler realized system: WGS84.TXT.
New realizations of WGS84 based on GPS data, such as G730, G873 and G1150. These new WGS84 realizations are coincident with ITRF at about 10-centimeter level.
For these realizations there are no official transformation parameters. This means that one can consider that ITRF coordinates are also expressed in WGS84 at 10 cm level.
However, the most recent G1674 realization adopted ITRF2008 coordinates for more than half of the reference stations and velocities of nearby sites for the others.
Thus, ITRF20014, ITRF2008 and WGS84 (G1674) are likely to agree at the centimeter level, yielding conventional 0-transformation parameters.

For more information on WGS84, ITRF and other (continental) datums such as NAD83 and ETRS89, see the page World Geodetic System 1984 (WGS84).

Transformation equations

The standard relation of transformation between two TRS's is an Euclidian similarity of seven parameters: three translation components, one delta scale factor, and three rotation angles,
designated respectively, T1, T2, T3, D, R1, R2, R3, and their first times derivations Ṫ1, Ṫ2, Ṫ3, Ḋ, Ṙ1, Ṙ2, Ṙ3.

The transformation of coordinate vector X₁, expressed in a reference system [1], into a coordinate vector X₂, expressed in a reference system [2], is given by the following equation:

X₂ = X₁ + T + D·X₁ + R·X₁ (1)

with:

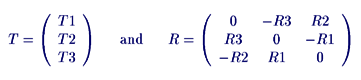
It is assumed that equation (1) is linear for sets of station coordinates provided by space geodetic technique (origin difference is about a few hundred meters, and differences in scale and orientation are of 10^-5 level).

Generally, X₁, X₂, T, D, R are functions of time, see equation (4). Differentiating equation (1) with respect to time gives:

Ẋ₂ = Ẋ₁ + Ṫ + Ḋ·X₁ + D·Ẋ₁ + Ṙ·X₁ + R·Ẋ₁ (2)

Parameters D and R are at the 10^-5 level and Ẋ is about 10 cm per year, so the terms D·Ẋ₁ and R·Ẋ₁ which represent about 0.1 mm over 100 years are negligible. Therefore, equation (2) could be writen as:

Ẋ₂ = Ẋ₁ + Ṫ + Ḋ·X₁ + Ṙ·X₁ (3)

On the other hand, for a given parameter P, its value at any epoch t is obtained by using equation:

P(t) = P(t₀) + Ṗ · (t – t₀) (4)

where t₀ is the reference epoch indicated in the transformation parameters table (e.g. 2005.0 for ITRF2008) and Ṗ is the rate of that parameter.

To transform between various ITRFyy realizations and other datums, it is necessary to take the sum of the incremental transformation parameters between the relevant ITRFyy realizations, all at epoch t.
These transformation parameters can then be added with those between ITRFyy and another datum such as NAD83 (CORS96), to give the full transformation from ITRFyy to NAD83 (CORS96) at epoch t.

Example: (ITRF2008 → NAD83 (CORS96)) = (ITRF2008 → ITRF2005) + (ITRF2005 → ITRF2000) + (ITRF2000 → ITRF97) + (ITRF97 → ITRF96) + (ITRF96 → NAD83 (CORS96))

Transformation parameters

Transformation parameters from ITRF2014 to past ITRFs are given in ITRF_Transformation_Parameters_.xlsx (ITRF sheet).

Rotations are for the position vector rotation convention. Units are meters, mas (milliarcsecons) and ppb (parts-per-billion).
1 mas = 0.001 " = 2.77778 e^-7 degrees = 4.84814 e^-9 radians. 0.001 " corresponds to about 0.030 m at the earth's surface.

Note. Not all transformation parameters in the ITRF sheet have been tested yet. Always check the original documentation.

International Terrestrial Reference Frame 2020 (ITRF2020)

In January 2019, the IERS disseminated a call for participation for a new ITRF2020 solution to be released by the ITRS Center in September or October 2021.

Six years of additional observations will become available at the end of 2020. New sites have been added to the ITRF network and new co-location sites and new local ties are now available. Also, the processing strategies of the individual techniques have improved and self-consistent reprocessed solutions are expected to be available. A rigorous Post-Seismic Deformation modeling of sites subject to major earthquakes will be operated, as for ITRF2014. Periodic signals observed in the station position time series will be modelled in order to estimate robust station velocities, and eventually combined at co-location sites. Nonlinear station motions caused by slow earthquakes or recent ice melting will need to be appropriately modelled.

More information can be found on the ITRF website, in the ITRF2020 Call for participation.

References

IOGP Geomatics Guidance Note 25 - Dynamic versus static CRSs and use of the ITRF
Reference Frames in Practice Manual - http://www.fig.net/pub/figpub/pub64/figpub64.htm
International Terrestrial Reference Frame - http://itrf.ensg.ign.fr/trans_para.php
European Terrestrial Reference Frame 89 - http://etrs89.ensg.ign.fr/memo-V8.pdf
North American Datum 1983 (2011) - http://www.ngs.noaa.gov/TOOLS/Htdp/Htdp.html
North American Datum 1983 (CSRS) - http://www.geod.nrcan.gc.ca/tools-outils/trnobs_e.php