How-to Deal with Mercator Projections
To ensure correct presentation of survey results using the various variants of the Mercator projection, please make sure to enter the projection settings correctly in the Qinsy Geodetic setup.
This page contains information from the following publications from the International Association of Oil & Gas Producers (IOGP).
Note that these documents are subject to revision, so make sure to check the latest versions on the IOGP website.
- IOGP Publication 373-07-2 – Coordinate conversions and transformation including formulas – April 2018
- IOGP Publication 373-23 – Geomatics Guidance Note 23 – Web Mercator – September 2016
On this page:
Mercator Projections
Mercator
EPSG Dataset coordinate operation method codes 9804, 9805 and 1044.
The standard Mercator projection is a map projection with the equator as the single standard parallel.
All other parallels of latitude are straight lines and the meridians are also straight lines at right angles to the equator, equally spaced.
It is the basis for the transverse and oblique forms of the projection (which are not discussed on this page).
It is little used for land mapping purposes but is in almost universal use for navigation charts.
As well as being conformal, i.e. angles on earth are preserved in the projection, it has the particular property that straight lines drawn on it are lines of constant bearing.
Thus navigators may derive their course from the angle the straight course line makes with the meridians.
Three variants of the Mercator projection are recognized, differentiated by the parameters used in the projection definition:
- Variant A, also known as Mercator (1SP) - The projection is defined with the equator as the single standard parallel, with scale factor on the equator also defined.
False grid coordinates are applied at the natural origin of the projection, the intersection of the equator and the longitude of origin. See figure below.
In the few cases in which the Mercator projection is used for terrestrial applications or land mapping, such as in Indonesia prior to the introduction of the Universal Transverse Mercator, this is the normal method of definition. - Variant B, defined through the latitude of two parallels equidistant either side of the equator upon which the grid scale is true - False grid coordinates are applied at the natural origin of the projection, the intersection of the equator and the longitude of origin. See figure below.
Variants A and B are alternative ways of defining the same projection – coordinates of a point will have the same values regardless of whether the definition is variant A or variant B. - Variant C, Mercator (2SP) defined through the latitude of two parallels equidistant either side of the equator upon which the grid scale is true, as in variant (B) - However, in variant C false grid coordinates are applied at a point other than the natural origin of the projection, called the false origin. See figure below.
If the values of the standard parallels and false grid coordinates are the same, coordinates for variant C will differ from those for variant B.
Variants A and B are special cases of the more general variant C. The defining parameters for the three Mercator variants are summarized in the following table:
| Mercator coordinate operation method | Variant A | Variant B | Variant C |
|---|---|---|---|
| EPSG Dataset code | 9804 | 9805 | 1044 |
| Standard parallels | 1 SP | 2 SPs | 2 SPs |
| Latitude of natural origin (φ0) | x (note 1) | ||
| Scale at natural origin (k0) | x | ||
| Latitude of standard parallel (φ1) | x (note 2) | x (note 2) | |
| Latitude of false origin (φF) | x | ||
| Longitude of natural origin (λ0) | x | x | x |
| False Easting / Easting at natural origin (FE) | x | x | |
| False Northing / Northing at natural origin (FN) | x | x | |
| Easting at false origin (EF) | x | ||
| Northing at false origin (NF) | x |
Notes:
1. In the formulas that follow the parameter latitude of natural origin (φ0) is not used. However for the Mercator variant A (1SP) method, for completeness in CRS labelling the EPSG Dataset includes this parameter, which must have a value of zero.
2. For each of variants B and C (the 2SP cases), there is a pair of standard parallels with equal latitude value but different signs. Only one needs to be defined.
Several equations are common between the variants:
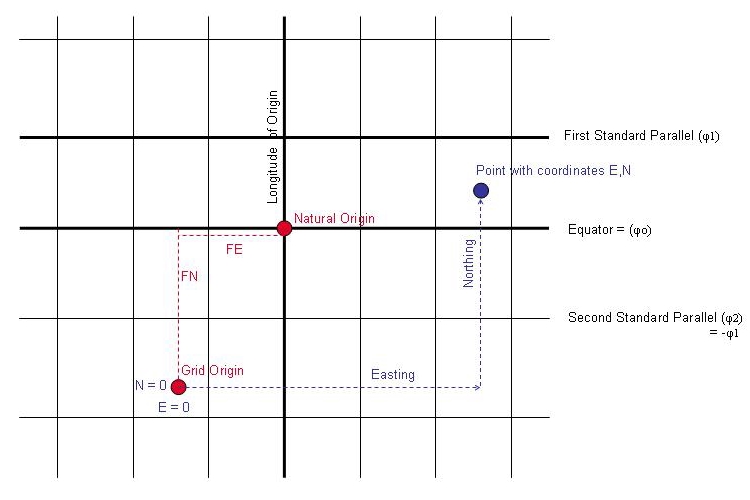
Figure – Mercator Variants A and B

Figure – Mercator Variant C
Mercator (Spherical)
EPSG Dataset coordinate operation method code 1026
The formulas to derive projected Easting and Northing coordinates from latitude and longitude using a sphere rather than ellipsoid as model of the earth where the radius of the sphere R will normally be one of the CRS parameters.
If the figure of the earth used is an ellipsoid rather than a sphere then R should be calculated as the radius of the conformal sphere at the projection origin at latitude φ0.
If latitude φ = 90º, Northing is infinite. The formula for Northing will fail near to the pole, and should not be used poleward of 88º.
Note that the parameter latitude of natural origin (φ0) is not used. However for the Merctor (Spherical) method, for completeness in CRS labelling the EPSG Dataset includes this parameter, which must have a value of zero.
Web Mercator (Pseudo-Mercator)
EPSG Dataset coordinate operation method code 1024
This method is utilised by some popular web mapping and visualisation applications such as Google Earth.
Strictly speaking the inclusion of 'Mercator' in the method name is misleading: it is not a Mercator projection, it is a different map projection and uses its own distinct formula: it is a separate method.
Unlike either the spherical or ellipsoidal Mercator projection methods, this method is not conformal: scale factor varies as a function of azimuth, which creates angular distortion.
Despite angular distortion there is no convergence in the meridian, so the graticule has a similar appearance to the graticule of a Mercator projection, but the graticules of the two projections do not overlay.
Points of Caution when using Web Mercator
It is impossible to project the curved surface of the model of the earth (usually an ellipsoid, sometimes a sphere) onto a flat plane without distortion.
Map projection methods are designed to preserve chosen properties, such as shape, area, or direction.
While geometric quantities (distances, areas, angles) derived from true Mercator can be corrected for this distortion, in Web Mercator they cannot. Therefore:
- Web Mercator coordinates should not be relied upon to derive metrics such as distances, areas, and directions from geographic features.
Web mapping applications such as Bing Maps, ArcGIS Online and Google Maps offer a very convenient background to give geographic context to data.
However, as is always the case when correctly merging data sets, it is critical that all data are in the same Coordinate Reference System.
- Before overlaying user data on Web Mercator maps, if not already on the WGS 84 CRS, the data must be referenced to or transformed to WGS 84.
- The correct Web Mercator map projection mathematics must be applied, or a positional error of up to 21 km can result. This can be a source of confusion as some applications have not clearly differentiated Web Mercator’s map projection method and formulas from true Mercator.
Some applications may support true Mercator but not Web Mercator: do not select “Mercator” as a substitute for Web Mercator. Additionally, make sure that the correct ellipsoid (WGS 84) is selected.
The Web Mercator mathematics are given in IOGP IOGP Publication 373-07-2 and are summarized in Appendix A of IOGP Publication 373-23.
Web Mercator is recommended as the CRS for the Common Operating Picture map viewer used in emergency response outside of polar regions because (a) most web map services are currently published in Web Mercator and (b) at the present time not all web mapping applications have the capability to transform published data to a user-defined CRS. Web Mercator provides a consistent framework for regional depiction of the incident and coordination of response operations. However, because projection distortions may be significant and cannot be corrected and because of the critical requirement for accurate and consistent reporting, Web Mercator should not be used to calculate critical metrics such as buffer distance, estimated spill area and volume, etc. Instead either “true” calculations on the ellipsoid or calculations in a specified and agreed projected CRS with a conformal (shape-preserving) map projection should be preferred. Ideally this will be the CRS in common use for large-scale mapping in the area, but care is needed to ensure that “common” must be understood by all parties involved.
In IOGP publication 373-23, the following conclusions and recommendations are provided:
- Web Mercator (the WGS 84 / Pseudo-Mercator CRS) can be selected for use just like any projected CRS, considering the context for its application.
- The Web Mercator’s map projection has different “projection-caused” distortions compared to the true Mercator map projection.
- Do not confuse Web Mercator with World Mercator or any other Mercator projection. In applications, do not select Mercator if Web Mercator is needed.
- Do not derive metrics such as distances, areas, or bearings / directions from Web Mercator coordinates. A projected CRS with a conformal (shapepreserving) map projection should be preferred.
- For emergency response Web Mercator may be used for regional mapping of the incident, but reiterating the previous bullet, it must not be relied upon for deriving accurate metrics.
When (if) coordinate transformation capability has become routinely available in all web map services, a more suitable CRS for COP operations may be chosen. - Use the EPSG names and codes in metadata, and provided formulas, parameters, and test points during implementation and application QC.
- Application developers should consider if an alternate to Web Mercator can be used for the base map and/or CRS of a published WMS service, in particular for applications at medium and large scales.
- For advice on the use of map projections consult a geomatics specialist.
Mercator Projections in Qinsy
In the current Qinsy Geodetic User Interface, the different variants of the Mercator Projection in the EPSG Database are all stored in the same sH0160 P2/94 record, which unfortunately only contains 4 or 5 fields - latitude, longitude, Easting, Northing, scale factor and Latitude of 1st standard parallel, but with a variant indication.
The Web Mercator is not supported because it is unqualified for surveying purposes. See the section above.
In the current Qinsy Geodetic User Interface, on the Setup Map Projection page in the 'Setup Coordinate Reference System' dialog, it is possible to select the Mercator projection type.
There is no default selected in the Geodetic User interface.
Note that using a scale factor of 1.0 for a non-zero standard parallel is equivalent to using a scale factor other than 1 for the zero latitude of origin (i.e. the equator).
Both scale factors as well as the standard parallel corresponding to the scale factor at the latitude of origin (usually the equator) are shown in the Qinsy Geodetic setup program.
It is a known issue that the P2/94 labels (fixed in the current user-interface as common denominator) do not reflect the actual EPSG naming convention.
For variants A and B, 'grid origin' should be read as 'natural origin' and for variant C, 'grid origin' should be read as 'false origin'. See the figures above.
Step 1 - Determine the Mercator Projection variant
Latitude of grid origin 0° N and Scale factor at latitude of origin not 1.0 -> EPSG Variant A
Latitude of grid origin 0° N and Latitude of standard parallel not 0° N -> EPSG Variant B
EPSG Variant A and EPSG Variant B are equivalent (i.e. we have two types of Variant C).
Latitude of grid origin not 0° N and Latitude of standard parallel not 0° N -> EPSG Variant C
Latitude of grid origin not 0° N and Scale factor at latitude of origin not 1.0 -> EPSG Variant C
Latitude of grid origin not 0° N and Scale factor at latitude of origin 1.0 -> old Qinsy Variant D
Note that the old (now deprecated) Qinsy Variant D looks like a subset of EPSG Variant A but actually leads to a different projection where the plane of projection is not perpendicular to the equator anymore (but tangent to the earth ellipsoid at the latitude of origin).
This was supported in Qinsy for some former offshore clients but is not supported in the EPGS Database so has been deprecated.
Step 2 - Enter the Mercator Projection parameters
Depending on the Mercator Projection variant, different sets of parameters must be entered.
The Latitude of grid origin is usually 0 (the equator). It is the latitude for which the Northing is given. Only for EPSG Variant C will this value be non-zero.
Tick the Standard parallel version box to enter the parameters for both EPSG Variant B and EPSG Variant C.
The following combinations of parameters are possible to enter the Mercator variants:

Figure – Qinsy setup for Mercator Variant A.
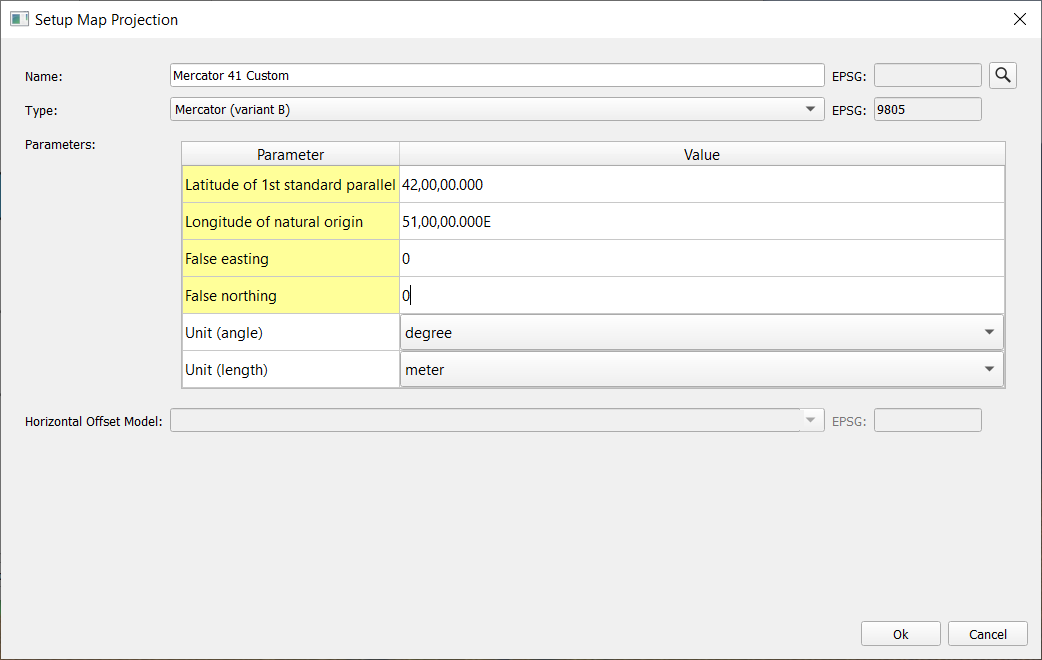
Figure – Qinsy setup for Mercator variant B.

Figure – Qinsy setup for Mercator Variant C.
