Geoid Model Glossary
On this page:
Geoid Model Introduction
Web Sites
References
- Ellipsoidally Referenced Surveying for Hydrography - http://www.fig.net/pub/figpub/pub62/figpub62.htm
Geoid Glossary
Geoid (W0): Specific equipotential (level) surface, which defines best, in a least-square sense, the global mean sea level. It is the true zero surface to measure elevations. For practical purpose, the geoid can also be defined as the equipotential surface representing a national vertical datum. (Unit: m2/s2)
Quasi-geoid: Surface parallel to the telluroid that is transferred to the mean sea level. The geoid and quasi-geoid are approximately the same surface over the oceans. However, the separation between the quasi-geoid and geoid can reach close to the metre level in mountainous areas. (Unit: m2/s2)
Equipotential Surface (W): It is a surface having a constant potential and being everywhere perpendicular to the direction of gravity. The equipotential surface is level, i.e., the water is at rest. It exists an infinity of equipotential surfaces. These surfaces do not intersect each other, but they converge towards the poles. Thus, the geometric distance between two equipotential surfaces is less at the poles than at the equator. Note: The mean sea level is not an equipotential surface because oceans have a permanent topography that is caused by temperature, salinity, currents, etc. (Unit: m2/s2)
Telluroid: Surface whose normal potential U is equal to the actual potential W at the Earth's surface along the ellipsoidal normal. The telluroid is not an equipotential surface. The telluroid was proposed by Molodenskii to avoid the complex determination of the topographical density and vertical gradient of gravity, which are necessary components in geoid modelling. (Unit: m2/s2)
Mean Sea Level (MSL): It is the arithmetic mean height of the sea in reference to a surface such as a chart datum, ellipsoid or geoid. It is determined from hourly observations over a 19-year cycle to average out the tidal lows and highs caused principally by the gravitational forces from the moon and sun. The mean sea level has small hills and valleys with respect to the geoid. It is the traditional zero elevation.
Vertical Datum: It is the reference surface for a height system, i.e., it is the zero elevation. The vertical datum is not necessarily an equipotential surface (e.g., CGVD28, ellipsoid and telluroid). A vertical datum is made of two components: a reference system and a reference frame. The former is its definition while the latter is its realization.
Geoid for Surveys
For decades now the geodetic community has been split down the middle over the question as to whether geoid or quasigeoid should be used as a reference surface for heights.
- The choice of the geoid implies that orthometric heights must be considered
- The choice of the quasi-geoid implies the use of the so-called normal heights.
The problem with the geoid, a physically meaningful surface, is that it is sensitive to the density variations within the Earth. The problem with the quasi-geoid, which is not a physically meaningful surface, is that it requires integration over the Earth's surface.
Geoid Model Glossary
Geoid Model: A geoid model is a mathematical representation of the geoid for a specific area, or for the whole earth. Software can use the geoid model to generate geoid separations for user’s points in a network.
Gravimetric Geoid Model: Geoid model that attempts to model the geoid as well as possible. No attempt is made to tie to an official vertical datum. Examples: USGG2009 in the USA and CGG2010 in Canada.
Hybrid Geoid Model: Geoid model that models the geoid but is also tied to an official vertical datum. Hybrid models convert between official h and H datums. Examples: GEOID09 in the USA and HTv2.0 in Canada.
Geoid Model for Surveys
Some countries, such as the USA and Canada, distribute both gravimetric and hybrid geoid models. Other countries only publish either gravimetric geoid models (e.g. GGM10 in Mexico) or hybrid geoid models (e.g. NLGEO2004 in The Netherlands).
- To conform with an official vertical datum or levelling network, use a hybrid geoid model.
- For proper water management at the highest precision, use a gravimetric geoid model.
- If the survey must conform with the elevations of nearby benchmarks or tide gauges,
(1) use the gravimetric geoid model to produce orthometric heights;
(2) do additional GPS at benchmarks or tide gauges and apply the gravimetric geoid model to determine the orthometric heights and the difference (or bias) between orthometric and published elevations (should be constant within a small area);
(3) apply this bias to the orthometric heights produced in step (1).
The last option can be done a lot quicker by taking the hybrid geoid model approach. Additional GPS observations at benchmarks are not required. This method, however, is less accurate over a large area, due to the inherent distortions in hybrid models.
Geoid Height Glossary
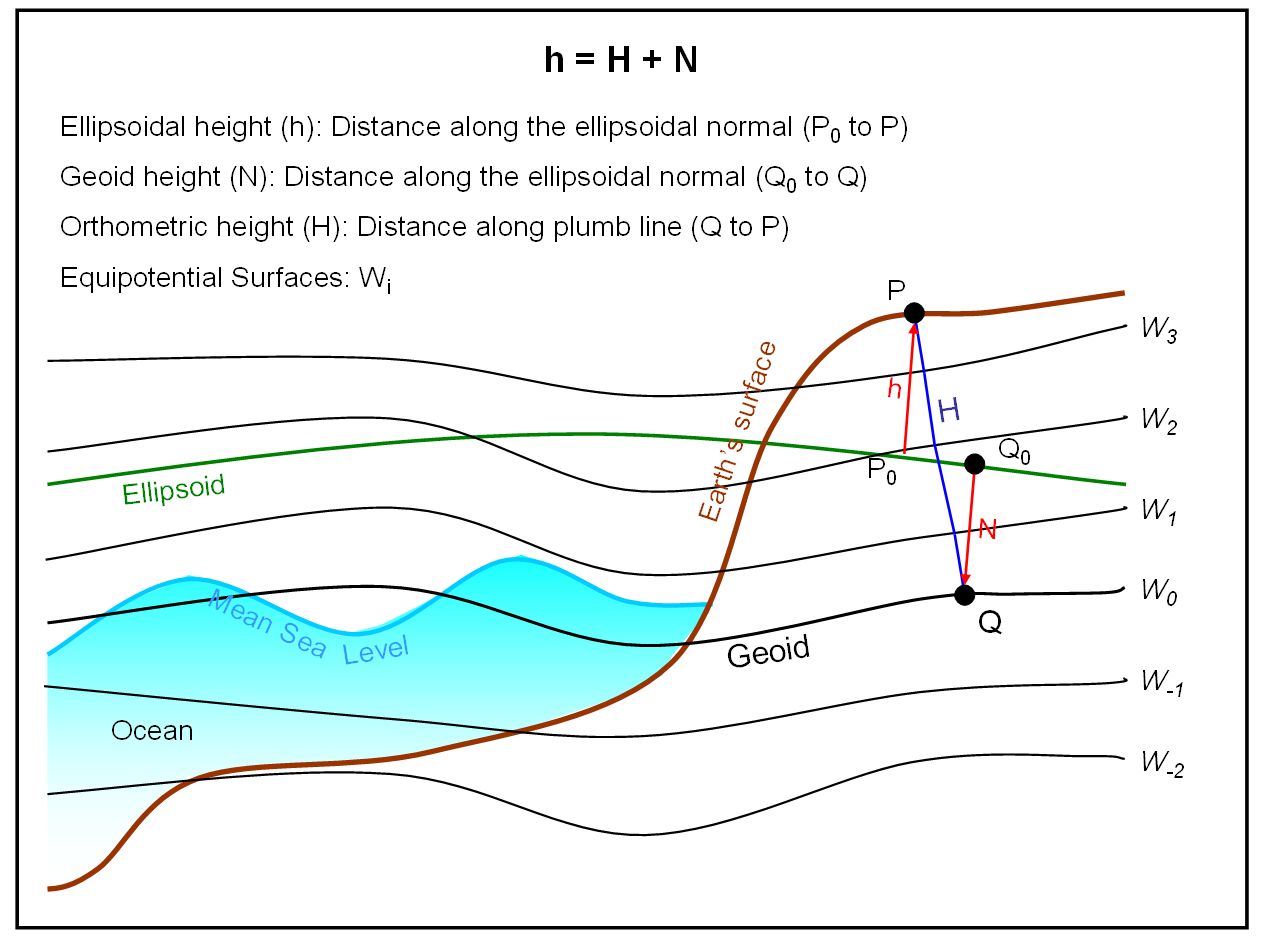
Ellipsoidal height (h): Elevation of a point above the reference ellipsoid. The distance is measured along the ellipsoidal normal. The ellipsoidal height is also called the geodetic height. (Unit: m)
Orthometric height (H): Elevation of a point above the geoid. It is measured along the plumb line, which is perpendicular to the equipotential surfaces. (Unit: m)
Normal Height (Hn): It is the elevation of a point above the quasi-geoid or elevation of the telluroid above the ellipsoid. The difference between normal and orthometric heights is more significant at high elevations. (Unit: m)
Geoid height (N): Separation between the reference ellipsoid (e.g., GRS80) and the geoid. The distance is measured along the ellipsoidal normal. Geoid heights are tied to a 3-D reference frame such as NAD83(CSRS) or ITRF. Geoid heights allow the conversion of ellipsoidal heights (h) to orthometric heights (H): H = h - N. The geoid height is also called the geoid undulation. (Unit: m)
Height anomaly (ζ): It is the separation between the telluroid and the earth or ocean surface. Also it can express the separation between the ellipsoid and the quasi-geoid. Height anomalies allow the conversion of ellipsoidal heights (h) to normal heights (Hn): H = h - ζ.(Unit: m)
Dynamic height (Hd): It is the potential difference between two equipotential surfaces along a plumb line scaled by a constant gravity value. For Canada and USA, the constant value is the normal gravity on the ellipsoid at latitude 45° (γ45°). Dynamic heights have no geometric meanings. They are mainly used for the management of large water basins (e.g., Great Lakes). The surface of a lake has a constant dynamic height because it is an equipotential surface. Because the equipotential surfaces converge towards the poles, the surface of the lake closer to a pole will have a lower orthometric height than the opposite end of the lake. (Unit: m)
Normal Orthometric height (Hno): This terminology is not proper, but it is used to define the type of heights currently available in Canada (CGVD28). These heights are neither orthometric nor normal, i.e., they are not compatible with the geoid or quasi-geoid. They are determined using normal gravity, but they are based on the formulation of orthometric heights. Normal orthometric heights are used in Canada because no actual gravity measurements were available at the time of the realization of CGVD28. The objective of the 1928 adjustment was the determination of the most accurate orthometric heights, which explains why they are commonly referred as orthometric heights. (Unit: m)
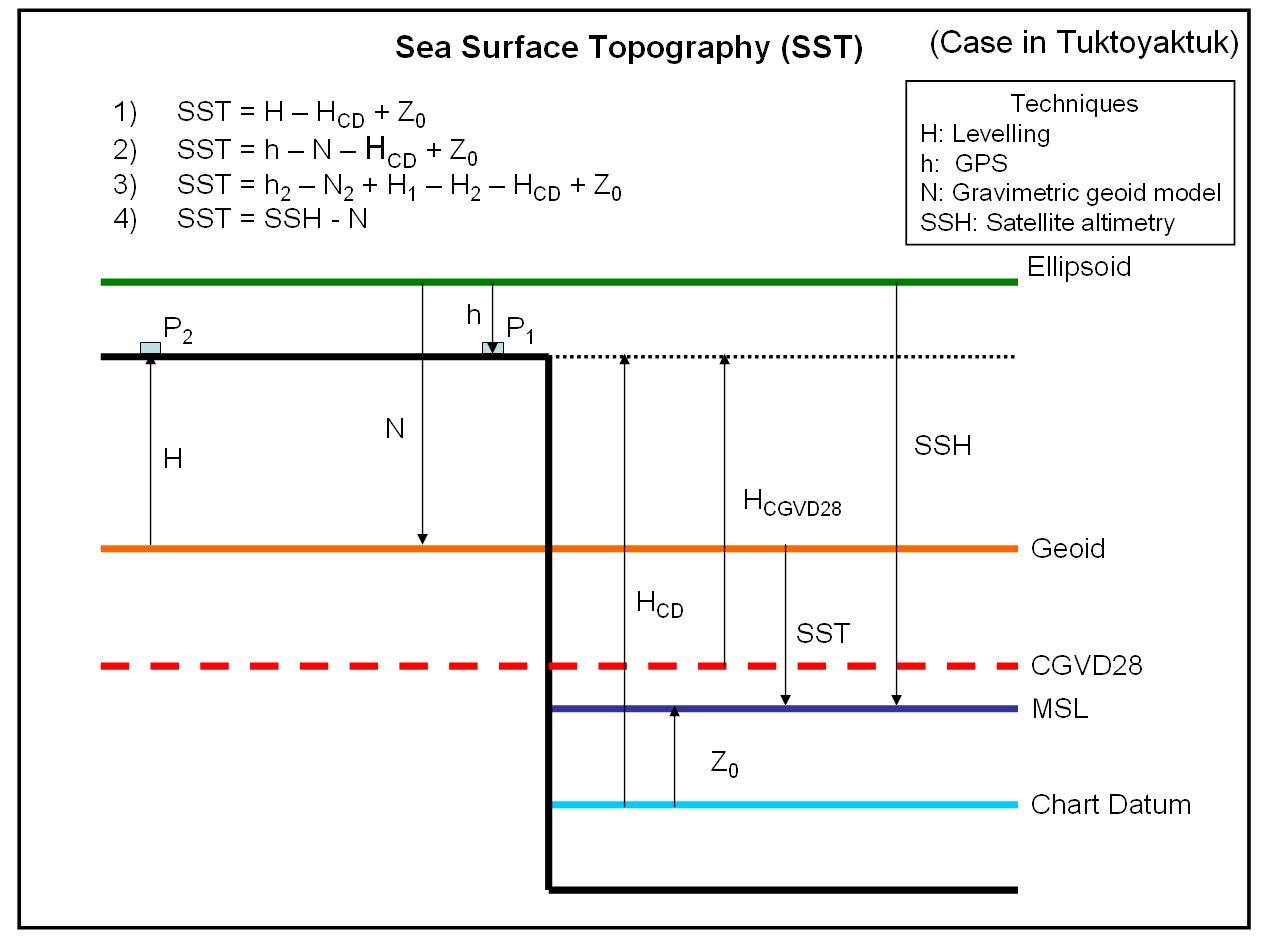
Sea Surface Height (SSH): It is the distance measured along the ellipsoidal normal between the ocean surface and ellipsoid. It is equivalent to the ellipsoidal height of the ocean surface. Instantaneous SSH can be observed by satellite radar altimetry (e.g., Topex/Poseidon, ERS-1, Jason, etc). (Unit: m)
Sea Surface Topography (SST): It is the separation between the geoid and ocean surface. SST can be determined from Sea Surface Height (SSH) measured from satellite radar altimetry and a geoid height (N): SST = SSH - N. SST is positive if the ocean surface is above the geoid. It is equivalent to the orthometric height of the ocean surface. (Unit: m)
Gravity (g): It is the combination of the gravitational (mass) and centrifugal (rotation) forces. Overall, gravity increases from ~9.78 m/s2 at the equator to ~9.83 m/s2 at the poles. The elevation and mass density are components that influenced the local gravity value. (Unit: m/s2 or Gal; 1 m/s2 = 100 Gal = 0.1 kGal = 1x105 mGal)
Normal gravity (γ): It is an approximate gravity value determined from the parameters defining an equipotential ellipsoid of revolution, e.g., GRS67, GRS80. (Unit: m/s2 or Gal; 1 m/s2 = 100 Gal = 0.1 kGal = 1x105 mGal)
Deflection of the vertical (ξ, η): It is the angle between the directions of the plumb line and ellipsoidal normal. It has two components: a north-south component (ξ) and an east-west component (η). (Unit: arcsec)
Geoid Heights for Surveys
The difference between the geoid and a quasi-geoid is that the geoid corresponds to a datum of orthometric height and the quasi-geoid to that of normal height. By considering the normal gravity gradient with respect to the surface of the mean reference ellipsoid, the quasi-geoid is defined as a function of the normal height In practice, when orthometric heights are used for determining the vertical datum, a quasi-geoid correction is applied to the fundamental formula of physical geodesy in order to accurately determine the geoid.
The relationship between the height anomaly ζ and the geoid undulation N can be expressed in terms of the topographic height H, the normal gravity γ, and the Bouguer anomaly ΔgB. The Bouguer anomaly term depends on the density of the terrain mass and the topography (height).
The difference between the geoid and the quasi-geoid is minute over moderate topographies, but it can reach several decimeters over high mountainous areas. Thus, the quasi-geoid correction cannot be ignored over rough terrains.